Geometry for Higher Spin Gravity: Conformal Structures, PDEs, and Q-manifolds
The idea is to bring together people studying mathematical aspects of higher- spin gravity, and experts on the several geometries which appeared in this construction, in particular conformal geometry, the geometry of partial differential equations, as well as differential graded geometry.
The construction of consistent interactions among gauge fields of spin higher than two is a notoriously difficult challenge. Each time some of the obstacles were overcome, various modern mathematical notions played a crucial role in the construction. Although higher-spin geometry in itself might presently remain somewhat elusive, various fundamental mathematical objects have already appeared in higher-spin gravity, originating from conformal geometry (Cartan connections, tractor bundles, Fefferman-Graham ambient metric, etc), the geometry of Partial Differential Equations (e.g. infinite jet bundles and their Cartan distributions), differential graded geometry (such as Q-manifolds, Batalin-Vilkovisky and Becchi-Rouet-Stora-Tyutin formalisms, Alexandrov-Kontsevich-Schwarz-Zaboronsky sigma models) and deformation quantization (for instance Fedosov-like connection, formality theorems, deformation theory, etc). The deep relation between higher-spin gravity and important topics of contemporary mathematics might keep some surprises in store and shed new light on each other. This promises fruitful exchanges from which both communities will benefit.
In addition to research talks by participants it is planned to have several introductory minicourses, including:
1st week (23/08-27/08):
I. Anderson, Introduction to the variational bicomplex
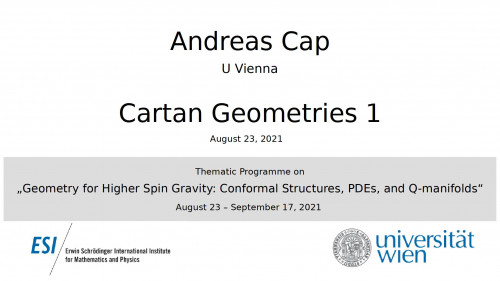
A. Cap, Cartan geometry
A. Cattaneo, An introduction to the BV-BFV formalism
E. Skvortsov, Conformal higher spin gravities and holography
2nd week (30/08-03/09):
M. Eastwood, Conformal differential geometry - the ambient metric and tractor connection
M. Henneaux, Introduction to asymptotic symmetries
A. Mikhailov, Q-manifolds and pure spinors
M. A. Vasiliev, Introduction to HS fields and interactions in AdS
3rd week (06/09-10/09):
T. Adamo, Twistors, ambitwistors and amplitudes
M. Eastwood, Conformal differential geometry - the ambient metric and tractor connection
J. Krasil'shchik, A. Verbovetsky, Geometry of PDEs: an overview
4th week (13/09-17/09):
G. Barnich, Photons and gravitons in a Casimir box
R. Gover, Boundary calculus
B. Kruglikov, Lie equations, Cartan bundles, Tanaka theory and differential invariants
Cartan Geometries 1
Conformal higher spin gravities and holography, 1
Self-dual Yang-Mills and self-dual gravity
Cartan geometries 2
Conformal higher spin gravities and holography, 2
Holography of new conformal higher spin theories in three dimensions
Maximally symmetric spacetimes and their lower dimensional theories
Introduction to exceptional field theory
(Higher-spin) gravity as a quantum effect on quantum space-time
Extracting Bigravity from String Amplitudes
Lecture 1: Variational Bicomplexes: The First Variational Formula.
Gauge theories with and without group actions and equivalences between them
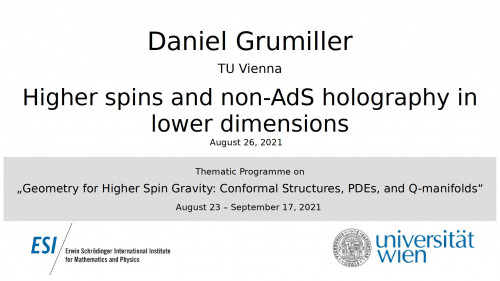
Higher spins and non-AdS holography in lower dimensions
Alberto Cattaneo (University of Zurich) An introduction to the BV-BFV formalism, 1
Lecture 2: Variational Bicomplexes: Diverse Applications
Alberto Cattaneo (University of Zurich) An introduction to the BV-BFV formalism, 2
Higher spin gravities and holography
Some constructions from graded geometry
NQ-manifolds and homotopy Lie bialgebroids
Introduction to HS fields and interactions in AdS I
(online)
Introduction to asymptotic symmetries - General formalism
A. Cattaneo (University of Zurich) An introduction to the BV-BFV formalism 3
Introduction to HS fields and interactions in AdS II
(online)
Introduction to asymptotic symmetries - Examples
BV-BFV Approach to General Relativity
Duality-invariant conformal higher-spin models
The BMS (super)algebra at spatial infinity
Lecture 1 : Classical phase space of bosonic string is a Q-manifold
(online)
Differential operators on bundles invariant with respect to compact operators
Recent results in higher-spin gauge theory
(online)
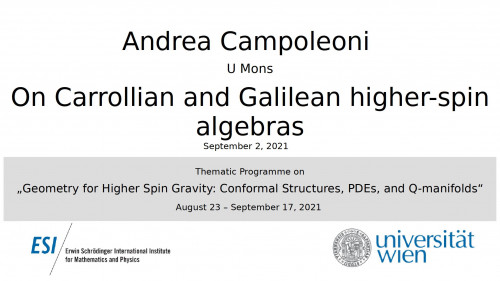
On Carrollian and Galilean higher-spin algebras
Lecture 2: Target space of pure spinor superstring is a Q-manifold
(online)
Conformal differential geometry - the ambient metric and tractor connection 1
Spinor-helicity formalism for higher-spin holography
Lecture 3: Normal form of nilpotent vector field near the tip of the pure spinor cone
(online)
Effective BV action for Chern-Simons theory on cylinders
(online)
Conformal differential geometry - the ambient metric and tractor connection 2
(online)
Lecture 1: Twistors and amplitudes
(online)
Democratic (N)ED
Calculus on Symplectic Manifolds
Lecture 2: Ambitwistors and amplitudes
(online)
Homotopy transfer for conserved currents and rigid symmetries in gauge theories
Lagrangian double copy to cubic order
Geometry of PDEs: an overview
(online)
Quantization of Contact Structures
(online)
TBA
Geometry of PDEs: an overview
(online)
Quantum field theory as a topological sigma.model
(online)
Non-regular parabolic geometries via the notion of bi-filtered manifolds
Lecture: Introduction to BMS symmetries and "asymptotic gravity vacua" from a geometric perspective
Geometry of PDEs: an overview
(online)
Informal extra lecture: Ambitwistors
(online)
Boundary calculus in conformal geometry. Lecture 1
Lecture 1: From finite temperature Casimir effect to massless scalar field partition functions
Calculi, cohomology, and Hopf algebroids
Boundary calculus in conformal geometry. Lecture 2
(online)
Lecture 2: Massless scalar field partition functions & real analytic Eisenstein series
Unfolding Conformal Geometry
(online)
Einstein-Weyl-like conditions and causal structures in dimension three
Higher conformal fundamental forms and the asymptotically Poincare-Einstein Condition
(online)
Lie equations, Cartan bundles, Tanaka theory and differential invariants 1
Algebraic classification of conformal invariants and conformal anomalies in arbitrary dimension
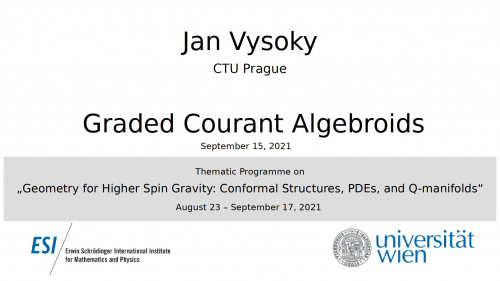
Graded Courant Algebroids
Lie equations, Cartan bundles, Tanaka theory and differential invariants 2
Lecture 3: Gravitons in a Casimir box
Tractor geometry of null-Infinity and gravitational radiations
Super-symmetry of geometric structures
Multiplicative Connections on Lie Groupoids
(online)
A mathematical overview of universal enveloping algebras and their universal properties
Organizers
| Name | Affiliation |
|---|---|
| Xavier Bekaert | University of Tours |
| Andreas Cap | University of Vienna |
| Stefan Fredenhagen | University of Vienna |
| Maxim Grigoriev | University of Mons |
| Alexei Kotov | University of Hradec Králové |
Attendees
| Name | Affiliation |
|---|---|
| Tim Adamo | University of Edinburgh |
| Konstantin Alkalaev | Lebedev Physical Institute & Lomonosov Moscow State University |
| Ian Anderson | Utah State University |
| Glenn Barnich | Free University of Brussels |
| Thomas Basile | University of Mons |
| Klaus Bering | Masaryk University |
| Nicolas Boulanger | University of Mons |
| Andrea Campoleoni | University of Mons |
| Alberto Cattaneo | University of Zurich |
| Luca Ciambelli | Free University of Brussels |
| Oscar Cosserat | University of La Rochelle |
| David De Filippi | University of Mons |
| Arnaud Delfante | University of Mons |
| Ivan Dneprov | Lomonosov Moscow State University |
| Laura Donnay | SISSA |
| Boris Doubrov | Belarusian State University |
| Cristina Draper Fontanals | Universidad de Malaga |
| Michael Eastwood | University of Adelaide |
| Dario Francia | Università Roma Tre |
| Jordan Francois | University of Mons |
| Jordi Frias | Lomonosov Moscow State University |
| Oscar Fuentealba | Free University of Brussels |
| Olga Gelfond | Lebedev Physical Institute |
| Rod Gover | University of Auckland |
| Vyacheslav Gritsaenko | Moscow Institute of Physics and Technology |
| Daniel Grumiller | Technical University of Vienna |
| Aliaksandr Hancharuk | University of Lyon |
| Christoph Harrach | University of Vienna |
| Marc Henneaux | Free University of Brussels |
| Yannick Herfray | Free University of Brussels |
| Daniel Hutchings | University of Western Australia |
| Carlo Iazeolla | Guglielmo Marconi University |
| Noriaki Ikeda | Ritsumeikan University |
| Euihun Joung | Kyung Hee University |
| Branislav Jurco | Charles University Prague |
| Igor Khavkine | Institute of Mathematics of the Czech Academy of Sciences |
| Yvette Kosmann-Schwarzbach | retired from Ecole polytechnique |
| Niels Kowalzig | University Federico II of Napoli |
| Joseph Krasil’shchik | Independent University of Moscow |
| Kirill Krasnov | University of Nottingham |
| Olaf Krüger | University of Vienna |
| Boris Kruglikov | University of Tromsø |
| Svatopluk Krysl | Charles University Prague |
| Sergei Kuzenko | University of Western Australia |
| Kristiansen Lara | Universidad de Chile |
| Camille Laurent-Gengoux | University of Lorraine |
| Rob Leigh | University of Illinois at Urbana-Champaign |
| Thomas Leistner | University of Adelaide |
| Iva Lovrekovic | Technical University of Vienna |
| Sucheta Majumdar | Free University of Brussels |
| Omid Makhmali | Polish Academy of Sciences |
| Chrysoula Markou | Max Planck Institute for Physics |
| Mikhail Markov | Lomonosov Moscow State University |
| Javier Matulich Fabres | Free University of Brussels |
| Ruslan Metsaev | Lebedev Physical Institute |
| Andrei Mikhailov | IFT UNESP |
| Nikita Misuna | Albert-Einstein-Institute |
| Karapet Mkrtchyan | Imperial College London |
| Pavel Mnev | University of Notre Dame du Lac |
| Kevin Morand | Sogang University |
| Timothy Moy | University of Adelaide |
| Turmoli Neogi | Free University of Brussels |
| Katharina Neusser | Masaryk University |
| Francisco José Palomo | Universidad de Malaga |
| Enrico Parisini | University of Southampton |
| Noémie Parrini | University of Mons |
| Mateo Paulišić | University of Rijeka |
| Simon Pekar | SISSA |
| Alfredo Perez | Centro de Estudios Cientificos |
| Michael Ponds | University of Western Australia |
| Dmitry Ponomarev | Lomonosov Moscow State University |
| Stefan Prohazka | University of Edinburgh |
| Nicolai Reshetikhin | University of California, Berkeley |
| Jan Rosseel | Ruđer Bošković Institute |
| Dmitrii Rudinskii | Lomonosov Moscow State University |
| Romain Ruzziconi | University of Oxford |
| Leonid Ryvkin | University of Göttingen |
| Ivo Sachs | Ludwig-Maximilians-University Munich |
| Katja Sagerschnig | Polish Academy of Sciences |
| Vladimir Salnikov | CNRS / U La Rochelle |
| Jakob Salzer | Harvard University |
| Henning Samtleben | Ecole Normale Superieure de Lyon |
| Andrea Santi | The Arctic University of Norway |
| Paolo Saracco | Free University of Brussels |
| Michele Schiavina | ETH Zürich |
| Pavel Sereda | Lomonosov Moscow State University |
| Alexey Sharapov | National Research Tomsk State University |
| Georgy Sharygin | Lomonosov Moscow State University |
| Ekaterina Shemyakova | University of Toledo |
| Eugene Skvortsov | University of Mons |
| Jan Slovak | Masaryk University |
| Harold Steinacker | University of Vienna |
| Thomas Strobl | University of Lyon |
| Per Sundell | Universidad Andrés Bello |
| Tom Sutherland | University of Vienna |
| Arman Taghavi-Chabert | University of Warsaw |
| Dennis The | University of Tromsø |
| Ricardo Troncoso | Centro de Estudios Cientificos |
| Arkady Tseytlin | Imperial College London |
| Mirian Tsulaia | Okinawa Institute of Science and Technology |
| Mikhail Vasiliev | Lebedev Physical Institute |
| Alexander Verbovetsky | Independent University of Moscow |
| Luca Vitagliano | University of Salerno |
| Alexander Voronov | University of Minnesota |
| Theodore Voronov | University of Manchester |
| Ivan Vuković | University of Rijeka |
| Jan Vysoky | Czech Technical University Prague |
| Andrew Waldron | University of California, Davis |
| Raphaela Wutte | Technical University of Vienna |
| Alexander Yan | Lomonosov Moscow State University |
| Yihao Yin | Nanjing University of Aeronautics and Astronautics |
| Lenka Zalabová | University of South Bohemia |

Aug. 24, 2021 11:00 — 12:00

Aug. 24, 2021 14:00 — 15:00

Aug. 24, 2021 15:30 — 16:30

Aug. 25, 2021 11:00 — 12:00

Aug. 25, 2021 14:00 — 15:00

Aug. 25, 2021 15:30 — 16:30

Aug. 26, 2021 09:30 — 10:30
Aug. 26, 2021 11:00 — 12:00

Aug. 26, 2021 15:30 — 16:30

Aug. 27, 2021 14:00 — 15:00

Aug. 27, 2021 15:30 — 16:30

Aug. 30, 2021 09:30 — 10:30

Aug. 30, 2021 11:00 — 12:00

Aug. 31, 2021 09:30 — 10:30

Aug. 31, 2021 11:00 — 12:00

Sept. 1, 2021 09:30 — 10:30

Sept. 1, 2021 11:00 — 12:00

Sept. 1, 2021 15:30 — 16:30

Sept. 2, 2021 09:30 — 10:30
Sept. 2, 2021 11:00 — 12:00

Sept. 3, 2021 09:30 — 10:30

Sept. 3, 2021 11:00 — 12:00

Sept. 3, 2021 15:30 — 16:30

Sept. 6, 2021 09:30 — 10:30

Sept. 7, 2021 14:00 — 15:00
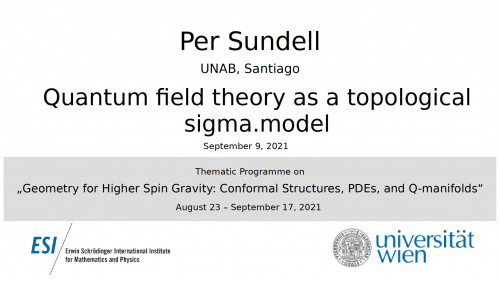
Sept. 9, 2021 15:30 — 16:30
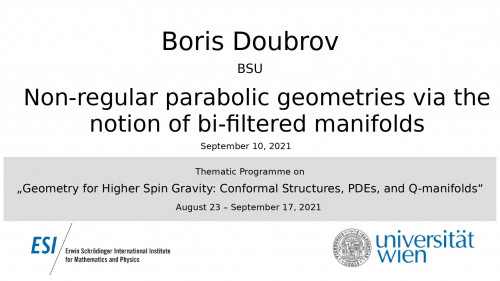
Sept. 10, 2021 09:30 — 10:30
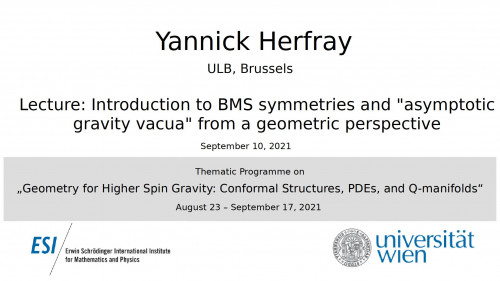
Sept. 10, 2021 11:00 — 12:00

Sept. 13, 2021 09:30 — 10:30
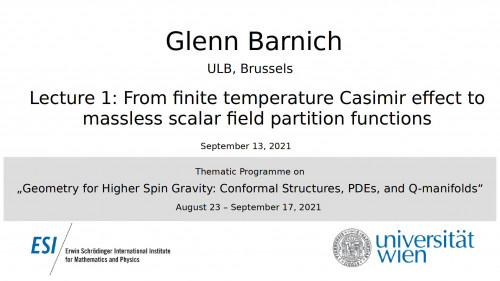
Sept. 13, 2021 11:00 — 12:00

Sept. 14, 2021 09:30 — 10:30
Sept. 14, 2021 11:00 — 12:00

Sept. 14, 2021 15:30 — 16:30

Sept. 15, 2021 09:30 — 10:30

Sept. 15, 2021 11:00 — 12:00

Sept. 15, 2021 14:00 — 15:00

Sept. 16, 2021 09:30 — 10:30

Sept. 16, 2021 14:00 — 15:00

Sept. 17, 2021 11:00 — 12:00

Sept. 17, 2021 14:00 — 15:00
- Type:
- Thematic Programme
- When:
- Aug. 23, 2021 — Sept. 17, 2021
- Where:
- ESI Boltzmann Lecture Hall
- Organizer(s):
-
Xavier Bekaert (U of Tours)
Andreas Cap (U of Vienna)
Stefan Fredenhagen (U of Vienna)
Maxim Grigoriev (U of Mons)
Alexei Kotov (U Hradec Králové)