Higher Structures and Field Theory - partially postponed
Important: Due to Covid-19 the first three weeks of the program have been shifted to online events.
The programme is devoted to the fruitful interaction between the theory of higher structures and mathematical approaches to field theory. This interaction goes in both directions.
On the one hand, field theory constantly inspires new developments in almost all fields of mathematics. And higher stuctures including the modern homotopy theory and supergeometry are among the first recepients of these ideas. There is also a renewed interest in mathematical approaches to field theory including algebraic perturbative quantum field theory, factorization homology etc.
On the other hand, many tools of the higher stucture theory naturally enter the language of quantum field theory. Among the examples are A-infinity and L-infinity stuctures, Batalin-Vilkovisky structures (first discovered in physics, they made their home in mathematics, and are now back on the physics arena) and many others. An impressive recent development is the use of higher structures in the classification of states of matter.
The programme will bring together specialists in higher structures and classical and quantum field theory to create more interaction on these exciting topics. Among the highlights of the programme are a focus week on the use of higher structures for a classification of states of, what is called, topological matter (during week 1), a workshop on higher structures (week 3), a focus week on the interaction between quantum field theory and higher structures (week 5), and a focus week on supergeometry and gauge theory (week 7). Among the programme events there will be mini-courses on perturbative algebraic quantum field theory, BV-methods, factorization algebras, and supergeometry (see below for a detailed description).
Overview:
Week 1 (August 3 - 7, 2020) Focus week on Topological Matter - online, have a look at "Associated Events"
Week 2 (August 10 - 14, 2020) Mini-course on The Poisson sigma model and integrable systems (N. Reshetikhin) - online, have a look at "Associated Events"
Week 3 (August 17 - 21, 2020) Workshop on Higher Structures - online, have a look at "Associated Events"
Week 4 (August 24 - 28, 2020) Mini-course on Advances in Algebraic Quantum Field Theory (K. Fredenhagen)
Week 5 (August 31 - September 4, 2020) Focus week on Higher Structures in Quantum field theory
Week 5 (August 31 - September 4, 2020) Mini-course on Higher structures in algebraic quantum field theory (A. Schenkel)
Week 6 (September 7 - 11, 2020) Mini-course on Courant algebroids, generalized Ricci flow, and T-duality (P. Severa)
Week 7 (September 14 - 18, 2020) Focus week on Supergeometry and Gauge Theory
Week 7 (September 14 - 18, 2020) Mini-course on Geometry of Q-manifolds and Gauge Theories (A. Kotov)
Week 8 (September 21 - 25, 2020) several talks and ESI Medal Award Ceremony
Details of the courses:
Week 2: N. Reshetikhin: The Poisson sigma model and integrable systems - ONLINE
The goal of the mini-course is to explain the relation between the semiclassical quantization of the Poisson sigma model and semiclassical amplitudes in quantum integrable systems.
Lecture 1: We recall the notion of quantum integrable systems and review the semiclassical asymptotics of joint eigenfunctions.
Lecture 2: For two integrable systems, we rewrite the semiclassical asymptotics of the scalar product of joint eigenfunctions of quantum integrals as a path integral of the Poisson sigma model with special boundary conditions.
Lecture 3: We will survey the BV-BFV quantization of the Poisson sigma model and its relation to the Kontsevich star product. This will then be used to find the semiclassical expansion to all orders of eigenfunctions of quantum integrable systems.
Week 4: K. Fredenhagen: Advances in Algebraic Quantum Field Theory - online
After an introduction into the formalism of algebraic quantum field theory recent developments will be discussed, in particular the relation to perturbative quantum field theory, to quantum gravity and to conceptual questions as entanglement and black hole evaporation.
Lecture 1: Framework of AQFT
Tuesday, August 25, 9:00 a.m.
Lecture 2: Perturbative approach towards AQFT
Thursday, August 27, 9:00 a.m.
Lecture 3: Perspectives and open problems
Friday, August 28, 9:00 a.m.
Slides of all three lectures by Prof. K. Fredenhagen
Recording of the lectures is available on our BigBlueButton link
Week 5: A. Schenkel: Higher structures in algebraic quantum field theory - online
Algebraic quantum field theory (AQFT) is a well-established framework to axiomatize and study quantum field theories on Lorentzian manifolds, i.e. spacetimes in the sense of Einstein’s theory of general relativity. In this mini course, I will give a brief introduction to AQFT and its recent higher categorical generalizations. This will cover the following topics:
Lecture 1: Operads and universal constructions in AQFT;
Monday, August 31, 2:00 p.m.
Slides of Lecture 1
Lecture 2: Local-to-global properties of quantum gauge theories;
Tuesday, September 1, 2:00 p.m.
Slides of Lecture 2
Lecture 3: Construction of simple examples by homological techniques. Throughout the course, I will introduce the relevant mathematical techniques from operad theory and homological algebra.
Thursday, September 3, 2:00 p.m.
Week 6: P. Severa: Courant algebroids, generalized Ricci flow, and T-duality
The aim of these lectures is to give an introduction to Courant algebroids (AKA generalized geometry), how they can be used to formulate T-duality known from String theory, and how their geometry implies that T-duality is compatible with the Ricci flow and with the string background equations. The main objects we shall study are Courant algebroids, their reductions and pullbacks, Dirac generating operators, and generalized metrics and the corresponding Ricci flows and Laplacians.
Lecture 1: Courant algebroids (CAs), classification of exact CAs, generalized metrics, pullbacks and reductions, Poisson-Lie T-duality
Monday, September 7, 2:00 p.m.
Lecture 2: divergences, generalized Ricci flow, generalized Laplacian, T-duality is compatible with the Ricci flow and the (bosonic or type I / heterotic) string background equations
Tuesday, September 8, 2:00 p.m.
Lecture 3: CAs in terms of symplectic dg manifolds and generating Dirac operators, T-duality and type II SUGRA
Thursday, September 10, 2:00 p.m.
Week 7: A. Kotov: Geometry of Q-manifolds and Gauge Theories
The focus of this mini-course lies on applications of differential graded (DG) geometry to gauge theories. The underlying grading of the dg manifolds (sometimes also called Q-manifolds) can be valued in Z,N, or Z_2, yielding different notions of super manifolds. Such a manifold is equipped with a degree one, and thus odd, vector field Q which squares to zero.
The gauge theories describe scalar fields coupled to a tower of differential form gauge fields. They are of a generalized Yang-Mills type (in the physically interesting cases) or of topological nature (like in the AKSZ model).
Lecture 1. Basics about the category of dg-manifolds with various important examples such as Lie algebras, Lie algebroids, and Lie infinity algebroids.
Monday, September 14, 10:00 a.m.
Lecture 2. We will introduce the notion of (compatible) G-structures on Q-manifolds. Furthermore, we will discuss characteristic classes in bundles in the category of Q-manifolds and their relation to the topological AKSZ sigma model, which generalizes important examples such as the Chern-Simons gauge theory or the Poisson sigma model to arbitrary dimensions.
Tuesday, September 15, 9:00 a.m.
Lecture 3. We show how to apply these techniques to the construction of Yang-Mills type higher gauge theories, with and without additional scalar fields. Particular attention will be paid to curved Yang-Mills-Higgs gauge theories, which constitute a non-trivial deformation of the gauge theoretical setting used in the current standard model of particle physics. While the standard theory of scalar fields coupled to 1-form gauge fields is governed by Lie groups or algebras and their representations, the more general theory is goverend by quadratic Lie algebroids, the geometry of which will be highligted in this course as well.
Thursday, September 17, 9:00 a.m.
Week 8: several talks and ESI Medal Award Ceremony
ESI Medal Award Ceremony
on September 24, 2020
- 14:00 Welcome
Prof. Christoph Dellago, ESI Director, University of Vienna - 14:05 Laudatio
Prof. Thomas Strobl, University of Lyon 1 - 14:45 Break
- 15:00 Award — ESI Medal
Prof. Christoph Dellago, ESI Director, University of Vienna - 15:15 Award Lecture
Prof. Anton Alekseev, University of Geneva - 16:00 Closing
Prof. Christoph Dellago, ESI Director, University of Vienna
Advances in Algebraic Quantum Field Theory I: Framework of AQFT
Topological quantum field theory with defects
Moduli spaces of flat connections as quasi-BV manifolds
Advances in Algebraic Quantum Field Theory II: Perturbative approach towards AQFT
Some topics in derived geometry motivated by the BV formalism
Advances in Algebraic Quantum Field Theory III: Perspectives and open problems
Higher structures in algebraic quantum field theory I
Shifted Symplectic Geometry and Derived Critical Loci.
Higher structures in algebraic quantum field theory II
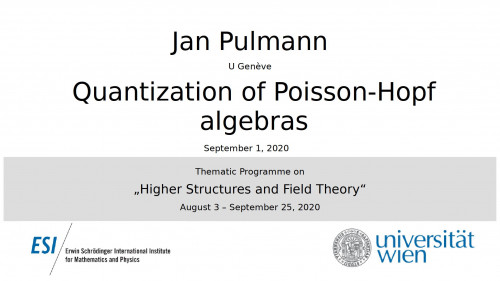
Quantization of Poisson-Hopf algebras
Fundamental group of quantum field theory
Dynamical zeta functions and topology
On the spectral problem of a three term difference operator.
Higher structures in algebraic quantum field theory III
Topological field theories with boundaries - some constructions and some applications
Deformations of Quantum Field Theories
Courant algebroids, generalized Ricci flow, and T-duality I
On the classification of Kontsevich formality maps
Higher-Spin Gauge Theory and Locality
BV and BFV for the H-twisted Poisson sigma model
Asymptotic fields in QFT from the point of view of BV-BFV formalism
Courant algebroids, generalized Ricci flow, and T-duality II
New integrable models from (non-commutative) deformation quantization
Dimensional reduction for Manin triples
Courant algebroids, generalized Ricci flow, and T-duality III
Quantum homotopy algebras and the homological perturabtion lemma.
Graded Manifolds: Some Issues
Geometry of Q-manifolds and Gauge Theories I
Exceptional field theory and exotic supergravity
Equivariant Poisson-Lie T-duality and supergravity
Geometry of Q-manifolds and Gauge Theories II
Duality Hierarchies
Presymplectic AKSZ form of Einstein gravity
Supergeometry and Applications
Geometry of Q-manifolds and Gauge Theories III
The higher supergeometry of the super-σ-model
Graded geometry for mixed-symmetry tensor gauge theories and duality
Q-manifolds, singular foliations, and singular leaves.
Multisymplectic (co-)momentum geometry
Higher Structures and Embedding tensors
The antifield-BRST approach to (gauge) field theories: an overview
Semiclassical quantization of Poisson structures: local symplectic groupoids, generating functions and the Poisson sigma model
- Online Workshop: Higher Structures and Field Theory - online Aug. 2, 2020 - Aug. 21, 2020
Organizers
| Name | Affiliation |
|---|---|
| Anton Alekseev | University of Genève |
| Stefan Fredenhagen | University of Vienna |
| Nicolai Reshetikhin | University of California, Berkeley |
| Thomas Strobl | University of Lyon |
| Chenchang Zhu | University of Göttingen |
Attendees
| Name | Affiliation |
|---|---|
| David Andriot | Technical University of Vienna |
| Mark Bugden | Mark Bugden |
| Nils Carqueville | University of Vienna |
| Athanasios Chatzistavrakidis | Ruđer Bošković Institute |
| Miquel Cueca | University of Göttingen |
| L Glaser | University of Vienna |
| Albin Grataloup | University of Montpellier |
| Aliaksandr Hancharuk | University of Lyon |
| Marc Henneaux | Free University of Brussels |
| Olaf Hohm | Humboldt University |
| Sergio Hörtner | University of Vienna |
| Nevena Ilieva | Bulgarian Academy of Science |
| Roberta Anna Iseppi | Georg-August-Universität |
| Larisa Jonke | Ruđer Bošković Institute |
| Branislav Jurco | Charles University Prague |
| Georgios Karagiannis | Ruđer Bošković Institute |
| Zoltan Kokenyesi | Ruđer Bošković Institute |
| Olaf Krüger | University of Vienna |
| Nima Moshayedi | University of Zurich |
| Damjan Pistalo | University of Zadar |
| Jan Pulmann | University of Genève |
| Jan Rosseel | Ruđer Bošković Institute |
| Dmitry Roytenberg | University of Amsterdam |
| Leonid Ryvkin | University of Duisburg-Essen |
| Vladimir Salnikov | University of La Rochelle |
| Henning Samtleben | Ecole Normale Superieure de Lyon |
| Pavol Severa | University of Genève |
| Eugene Skvortsov | University of Mons |
| Harold Steinacker | University of Vienna |
| Rafał R. Suszek | University of Warsaw |
| Fridrich Valach | Imperial College London |
| David Vergeiner | University of Vienna |
| Cornelia Vizman | West University of Timisoara |
| Jan Vysoky | Czech Technical University Prague |

Aug. 25, 2020 09:00 — 10:00

Aug. 26, 2020 15:30 — 16:30

Aug. 27, 2020 09:00 — 10:00
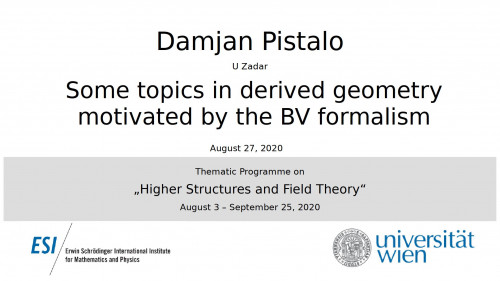
Aug. 27, 2020 11:00 — 12:00

Aug. 28, 2020 09:00 — 10:00

Aug. 31, 2020 14:00 — 15:00

Sept. 1, 2020 14:00 — 15:00

Sept. 2, 2020 14:00 — 14:45

Sept. 3, 2020 14:00 — 15:00

Sept. 3, 2020 15:30 — 16:15
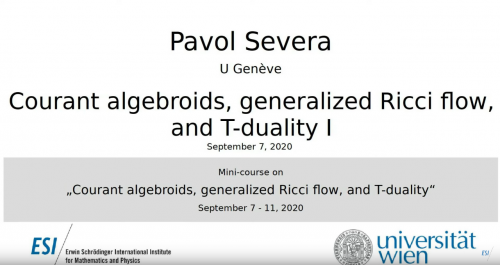
Sept. 7, 2020 14:00 — 15:00

Sept. 7, 2020 15:30 — 16:15
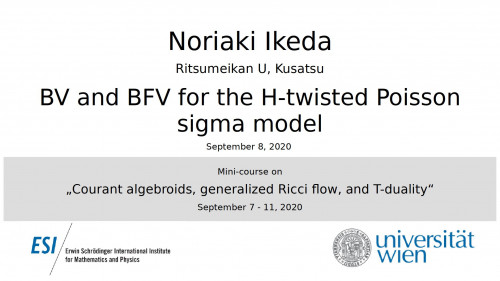
Sept. 8, 2020 11:00 — 11:45
Sept. 8, 2020 11:45 — 12:30
Sept. 8, 2020 14:00 — 15:00
Sept. 8, 2020 15:30 — 16:30
Sept. 10, 2020 14:00 — 15:00

Sept. 10, 2020 15:30 — 16:30

Sept. 14, 2020 14:00 — 15:00

Sept. 14, 2020 15:30 — 16:30

Sept. 16, 2020 14:00 — 14:40

Sept. 17, 2020 14:00 — 15:00

Sept. 17, 2020 15:30 — 16:30

Sept. 18, 2020 11:00 — 11:40

Sept. 21, 2020 14:00 — 14:40

Sept. 23, 2020 15:00 — 15:40
- Type:
- Thematic Programme
- When:
- Aug. 3, 2020 — Sept. 25, 2020
- Where:
- ESI Boltzmann Lecture Hall
- Organizer(s):
-
Anton Alekseev (U Genève)
Stefan Fredenhagen (U of Vienna)
Nicolai Reshetikhin (UC, Berkeley)
Thomas Strobl (U Lyon)
Chenchang Zhu (U Göttingen)